Calculating Capacitor Charge/Discharge Time using RC Constant

Swagatam

Capacitor charge and discharge periods is usually calculated through an RC constant called tau, expressed as the product of R and C, where C is the capacitance and R is the resistance parameter that may be in series or parallel with the capacitor C. It may be expressed as shown below:

τ = R C

The RC constant tau may be defined as the period required to charge a given capacitor through an associated series resistor by a difference of approximately 63.2% between its initial charge level and the final charge level.

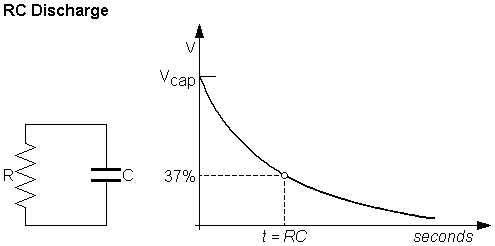
Conversely, the above expressed RC constant may be defined as the period required to discharge the same capacitor through a parallel resistor until 36.8% of the charge level is left.

The reason behind setting these limits is the extremely sluggish response by the capacitor beyond these limits which causes the charging or discharging processes to almost take an infinite amount of time to reach the respective full charge or full discharge levels, and therefore is ignored in the formula.

The value of tau is derived from the mathematical constant e, or

$<img decoding="async" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/c353c6664fc19884c569db1191a9d08365252592" alt="1-e^{{-1}}" width="3194.3" height="1223.9">$ ,

and to be more precise this may be expressed as the voltage required for charging the capacitor with respect to the parameter "time", as indicated below:

Charging

V(t) = V0(1−e^−t/τ)

Discharging

V(t) = V0(e^−t/τ)

Cutoff frequency

The time constant

$<img decoding="async" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/38a7dcde9730ef0853809fefc18d88771f95206c" alt="tau " width="517.5" height="721.6">$ is also typically associated with an alternative parameter, the cutoff frequency fc, and may be expressed by the formula:

τ = R C = 1/ 2 π f c

rearranging the above gives:, f c = 1 / 2 π R C = 1/ 2 π τ

where resistance in ohms and capacitance in farads yields the time constant in seconds or the frequency in Hz.

The above expressions may be further understood with short conditional equations, for example:

fc in Hz = 159155 / τ in µsτ in µs = 159155 / fc in Hz

Other similar useful equations are represented below which can be used for assessing a typical RC constant behavior:
rise time (20% to 80%)

t r ≈ 1.4 τ ≈ 0.22 / f c

rise time (10% to 90%)

t r ≈ 2.2 τ ≈ 0.35 / f c

In certain complicated circuits that may accompany in excess of one resistor and/or capacitor, the open-circuit time constant approach happens to offer a way of deriving the cutoff frequency by analyzing and calculating the total of many associated RC time constants.