In this article I have explained a simple yet accurate, wide range inductance meter circuit. The design utilizes only transistors as the main active components and a handful of inexpensive passive components.
The proposed Inductance meter circuit can measure inductance or coil values accurately over the given ranges and as a bonus the circuit is also capable of measuring the complementary capacitor values as accurately.
Circuit Operation
The circuit functioning may be understood with the following points:
As we all know that inductors are fundamentally related to generating frequencies or in other words with pulsating or AC supplies.
Therefore for measuring such components we need to force them with their specific functions in order to enable extraction of their hidden characteristics or attributes.
Here the coil in question is forced to oscillate at a given frequency, and since this frequency depends on the L value of the particular inductor becomes measurable through an analogue device such as a moving coil meter after suitably converting the frequency into amplified voltage/current.
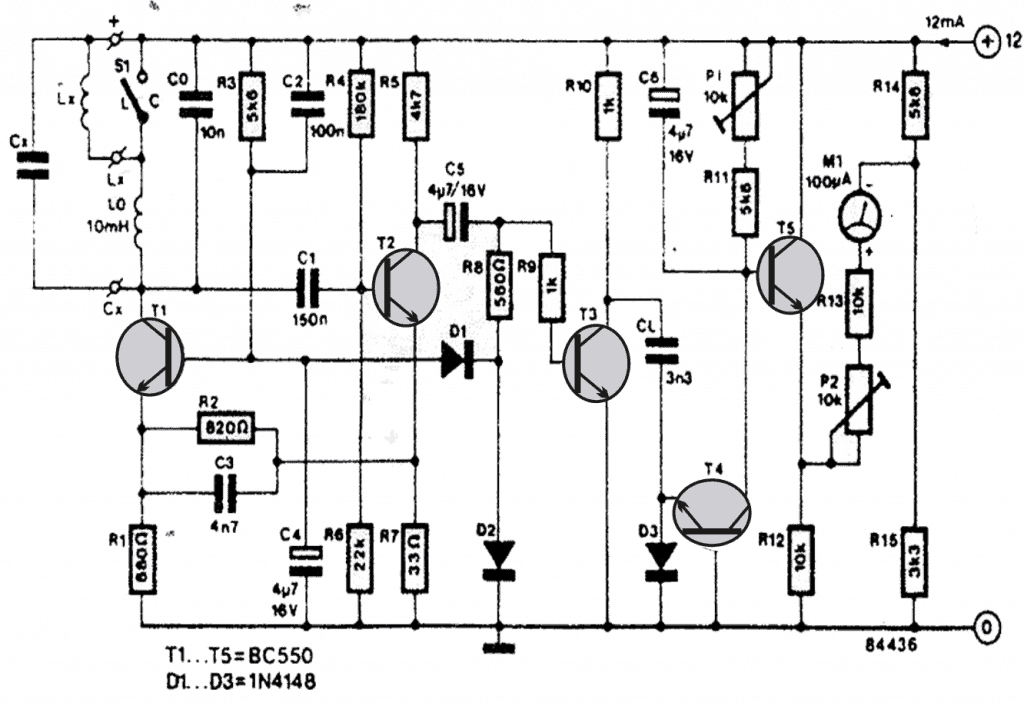
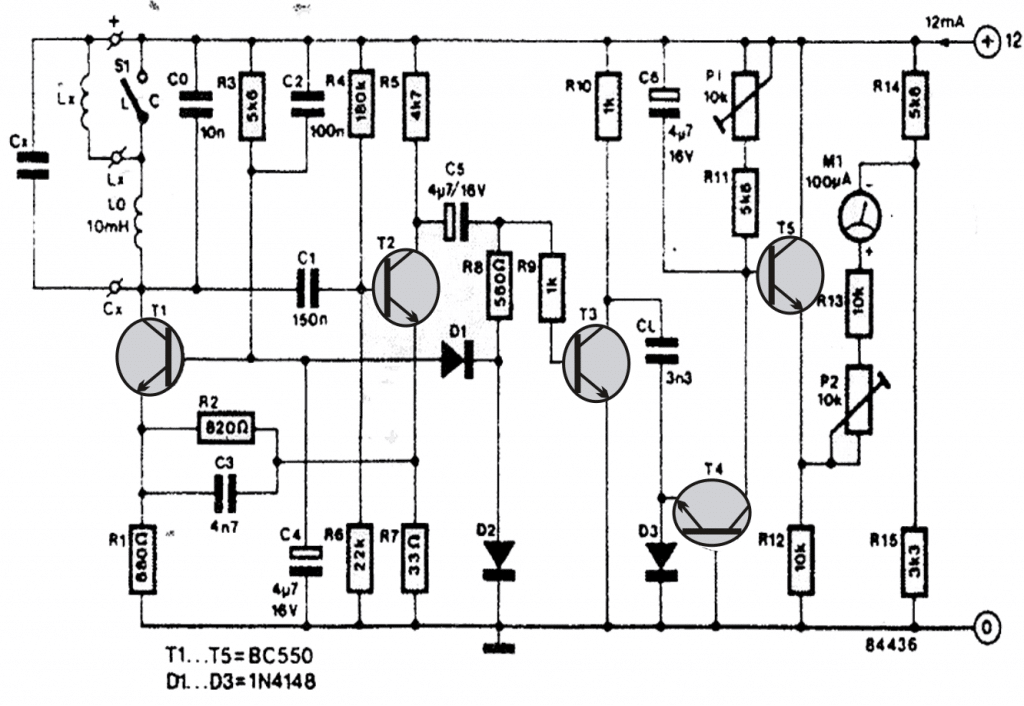
In the shown inductance meter circuit, T1 along Lo, Lx, Co, Cx together forms a Colpitts oscillator type of self oscillating configuration, whose frequency is directly determined by the above L and C components.
Transistor T2 and the associated parts help amplifying the generated pulses at the collector of T1 to reasonable potentials which is fed to the next stage comprising T4/T5 for further processing.
The T4/T5 stage raise the current and integrate the acquired info to appreciable levels so that it becomes readable over the connected uA meter.
Range Selection Option
Here Cx and Co basically provides the range selection option, many good quality caps with precise values may be positioned in the slot, with a provision of selecting the desired one via a rotary switch. This will allow an instant selecting facility of any desired range for enabling wider measurement of any particular inductor.
Conversely, correctly measured inductors/capacitor may be positioned at Co, Lo and Lx for getting an equivalent meter deflections for any unknown capacitor at Cx.
P1 and P2 may be used for monitoring and adjusting the zero position of the meter, it also allows fine tuning of the selected range over the meter.
Meter FSD calibration can be achieved by using the formula:
ni = nm(1 – fr)/(1 – fc)
where ni is the number of divisions measured on the scale, nm = total number of division of the scale, fr = relative frequency, fc = the smallest relative frequency measured.
The current consumption would be around 12mA at 12V while an inductor is being measured.
Circuit Diagram