In this article I have explained how to calculate resistor and capacitor values in transformerless power supply circuits using simple formulas like ohms law.
Analyzing a Capactive Power Supply
Before I have explained the formula for calculating and optimizing resistor and capacitor values in a transformerless power supply, it would be important to first summarize a standard transformerless power supply design.
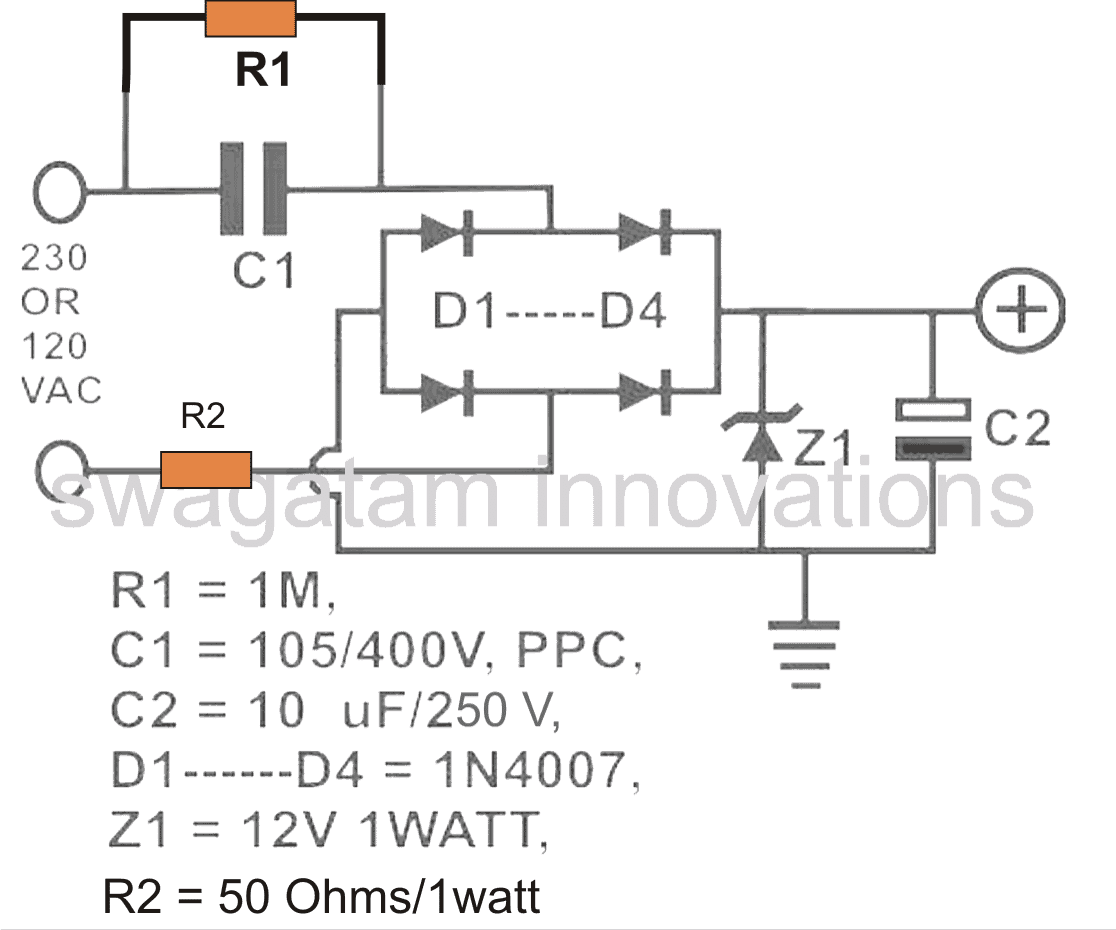
Referring to the diagram, the various components involved are assigned with the following specific functions:
C1 is the nonopolar high voltage capacitor which is introduced for dropping the lethal mains current to the desired limits as per the load specification.
This component thus becomes extremely crucial due to the assigned mains current limiting function.
D1 to D4 are configured as a bridge rectifier network for rectifying the stepped down AC from C1, in order to make the output suitable to any intended DC load.
Z1 is positioned for stabilizing the output to the required safe voltage limits.
C2 is installed to filter out any ripple in the DC and to create a perfectly clean DC for the connected load.
R2 may be optional but is recommended for tackling a switch ON surge from mains, although preferably this component must be replaced with a NTC thermistor.
Using Ohm's Law
We all know how Ohm’s law works and how to use it for finding the unknown parameter when the other two are known.
However, with a capacitive type of power supply having peculiar features and with LEDs connected to it, calculating current, voltage drop and LED resistor becomes a bit confusing.
How to Calculate and Deduce Current, Voltage Parameters in Transformerless Power Supplies.
After carefully studying the relevant patterns, I devised a simple and effective way of solving the above issues, especially when the power supply used is a transformerless one or incorporates PPC capacitors or reactance for controlling current.
Evaluating Current in Capacitive Power Supplies
Typically, a transformerless power supply will produce an output with very low current values but with voltages equal to the applied AC mains (until it’s loaded).
For example, a 1 µF, 400 V (breakdown voltage) when connected to a 220 V x 1.4 = 308V (after bridge) mains supply will produce a maximum of 70 mA of current and an initial voltage reading of 308 Volts.
However this voltage will show a very linear drop as the output gets loaded and current is drawn from the “70 mA” reservoir.

We know that if the load consumes the whole 70 mA would mean the voltage dropping to almost zero.
Now since this drop is linear, we can simply divide the initial output voltage with the max current to find the voltage drops that would occur for different magnitudes of load currents.
Therefore dividing 308 volts by 70 mA gives 4.4V. This is the rate at which the voltage will drop for every 1 mA of current added with the load.
That implies if the load consumes 20 mA of current, the drop in voltage will be 20 × 4.4 = 88 volts, so the output now will show a voltage of 308 – 62.8 = 220 volts DC(after bridge).
For example with a 1 watt LED connected directly to this circuit without a resistor would show a voltage equal to forward voltage drop of the LED (3.3V), this is because the LED is sinking almost all the current available from the capacitor.
However the voltage across the LED is not dropping to zero because the forward voltage is maximum specified voltage that can drop across it.
From the above discussion and analysis, it becomes clear that voltage in any power supply unit is immaterial if the current delivering capability of the power supply is "relatively" low.
For example if we consider an LED, it can withstand 30 to 40 mA current at voltages close to its "forward voltage drop", however at higher voltages this current can become dangerous for the LED, so it's all about keeping the maximum current equal to the maximum safe tolerable limit of the load.
Calculating Resistor Values
Resistor for the Load: When an LED is used as the load, it is recommended to choose a capacitor whose reactance value allows only the maximum tolerable current to the LED, in which case a resistor can be totally avoided.
If the capacitor value is large with higher current outputs, then probably as discussed above we can incorporate a resistor to reduce the current to tolerable limits.
Calculating Surge Limit Resistor: The resistor R2 in the above diagram forms is included as the switch-ON surge limiter resistor. It basically protects the vulnerable load from the initial surge current.
During the initial switch ON periods, the capacitor C1 acts like a complete short circuit, although just for a few milliseconds, and may allow the whole 220V across the output.
This may be enough to blow the sensitive electronic circuits or LEDs connected with the supply, which also includes the stabilizing zener diode.
Since the zener diode forms the first electronic device in line which needs to be safeguarded from the initial surge, R2 can be calculated as per the zener diode specifications, and maximum zener current, or zener dissipation.
The maximum tolerable current by the zener for our example will be 1 watt / 12 V = 0.083 amps.
Therefore R2 should be = 12 / 0.083 = 144 Ohms
However, since the surge current is only for a milliseconds, this value could be much lower than this.
Here. we are not considering the 310V input for the zener calculation, since the current is limited to 70 mA by the C1.
Since R2 can unnecessarily restrict precious current for the load during the normal operations, it must be ideally an NTC type of resistor.
An NTC will make sure that the current is restricted only during the initial switch ON period, and then the full 70 mA is allowed to pass unrestricted for the load.
Calculating the Discharge Resistor: Resistor R1 is used for discharging the stored high voltage charge inside C1, whenever the circuit is unplugged from the mains.
R1 value should be as low a possible for fast discharging of C1, yet dissipate minimum heat while being connected with the mains AC.
Since R1 can be a 1/4 watt resistor, its dissipation must be lower than 0.25 / 310 = 0.0008 amps or 0.8 mA.
Therefore R1 = 310 / 0.0008 = 387500 Ohms or 390 k approximately.
Calculating a 20 mA LED Resistor
Example: In the shown diagram, the value of the capacitor produces 70 mA of max. current which is quite high for any LED to withstand. Using the standard LED/resistor formula:
R = (supply voltage VS – LED forward voltage VF) / LED current IL,
= (220 - 3.3)/0.02 = 10.83K,
However the 10.83K value looks pretty huge, and would substantially drop the illumination on the LED....none-the-less the calculations look absolutely legitimate....so are we missing something here??
I think here the voltage "220" might not be correct because ultimately the LED would be requiring just 3.3V....so why not apply this value in the above formula and check the results? In case you have used a zener diode, then the zener value could be applied here instead.
Ok, here we go again.
R = 3.3/0.02 = 165 ohms
Now this looks much better.
In case you used, let's say a 12V zener diode before the LED, the formula could be calculated as given below:
R = (supply voltage VS – LED forward voltage VF) / LED current IL,
= (12 - 3.3)/0.02 = 435 Ohms,
Therefore the value of the resistor for controlling one red LED safely would be around 400 ohm.
Finding Capacitor Current
In the entire transformerless design discussed above, C1 is the one crucial component which must be dimensioned correctly so that the current output from it is optimized optimally as per the load specification.
Selecting a high value capacitor for a relatively smaller load may increase the risk of excessive surge current entering the load and damaging it sooner.
A properly calculated capacitor on the contrary ensures a controlled surge inrush and nominal dissipation maintaining adequate safety for the connected load.
Using Ohm's Law
The magnitude of current that may be optimally permissible through a transformerless power supply for a particular load may be calculated by using Ohm's law:
I = V/R
where I = current, V = Voltage, R = Resistance
However as we can see, in the above formula R is an odd parameter since we are dealing with a capacitor as the current limiting member.
In order to crack this we need to derive a method which will translate the capacitor's current limiting value in terms of Ohms or resistance unit, so that the Ohm's law formula could be solved.
Calculating Capacitor Reactance
To do this we first find out the reactance of the capacitor which may be considered as the resistance equivalent of a resistor.
The formula for reactance is:
Xc = 1/2(pi) fC
where Xc = reactance,
pi = 22/7
f = frequency
C = capacitor value in Farads
The result obtained from the above formula is in Ohms which can be directly substituted in our previously mentioned Ohm's law.
Let's solve an example for understanding the implementation of the above formulas:
Let's see how much current a 1uF capacitor can deliver to a particular load:
We have the following data in our hand:
pi = 22/7 = 3.14
f = 50 Hz (mains AC frequency)
and C= 1uF or 0.000001F
Solving the reactance equation using the above data gives:
Xc = 1 / (2 x 3.14 x 50 x 0.000001)
= 3184 ohms approximately
Substituting this equivalent resistance value in our Ohm's law formula, we get:
R = V/I
or I = V/R
Assuming V = 220V (since the capacitor is intended to work with the mains voltage.)
We get:
I = 220/3184
= 0.069 amps or 69 mA approximately
Similarly other capacitors can be calculated for knowing their maximum current delivering capacity or rating.
The above discussion comprehensively explains how a capacitor current may be calculated in any relevant circuit, particularly in transformerless capacitive power supplies.
WARNING: THE ABOVE DESIGN IS NOT ISOLATED FROM MAINS INPUT, THEREFORE THE WHOLE UNIT COULD BE FLOATING WITH LETHAL INPUT MAINS, BE EXTREMELY CAREFUL WHILE HANDLING IN SWITCHED ON POSITION.

Have Questions? Please Leave a Comment. I have answered over 50,000. Kindly ensure the comments are related to the above topic.
Very good and useful analysis for free ,Keep it up sir.I have made all efforts to repair damaged common china rechargeable touch on charging circuit,all to failure.With this analysis and other elsewhere,i made a breakthrough.But its good to go for a capacitor with 8 or 10volts so that if the led indicator drops some volts,there will be enough to charge the 4 or 5v battery.
But why is it that when you replaced a burnt component in a circuit like the rechargeable touch,still it wont work.
Thanks so much.
Thank you Patrick,
There may be some other components which are faulty, that is why the device is not working. You may have check each and every component to diagnose the unit fully.
Many many thanks swagatam for this page
Regards
Rajat Kumar Sardar
Dip IETE
Thank you Rajat, Glad it helped you.
HI
Guys….
Step 1 = Requirement is 5v o/p and load current is 120 to 150mA
Step 2 = I already try for this circuit given data is c1 = 2.2uF R1= 1M ohm R2 = 110 ohm 2W & 5W ( resistor is heated ) why it is heated i dont know give solution for that R2 resistor
Step 3 = after bridge i am using zener diode 16.5v and across C2 = 1uf 65v
Step 4 = after that zener diode o/p was given in to the i/p 7805 voltage regulator
Step 5 = (I=V/R) V=5V & Load is 33ohm
I= V/R 5/33Ohm
I = 150mA current but i didnot get 150mA I got only 110mA output current but that time LDO and zenor diode accros volt is dip around vz= 11.5v ldo =3.9v why ? give me any solution plz….?
You cannot stop the heat generation, because you are trying to drop 300V to 16V through a linear regulator. The heat can be reduced only if a zero crossing controlled circuit is used, as explained in the last concept of this article.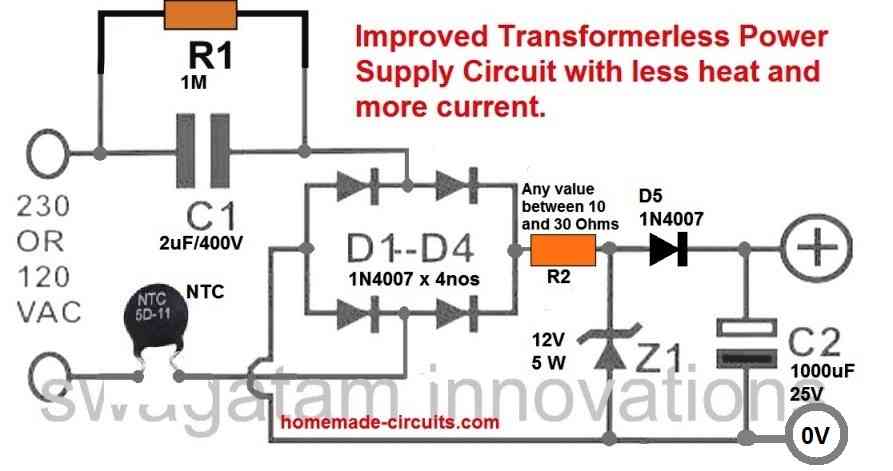
Still however you can try the following modifications in your circuit and check how it works.
Thanks for your useful topics. The max power consumption of such a circuit with C1=0.1uF will be Pmax= 0.069A x 220Vrms= 15.18W, is that correct?
That is correct!
Hello:
?R2 should be=(308-12)V/0.083A=
=3.56K?
Please, your clarification
Regards
R2 is included only to control the initial switch ON surge….it is not a permanent current limiting resistor. The input capacitor will itself control the current to the desired limit.
Any value between 50 ohm and 100 ohm should work.
Alternatively you cn use a 10 ohm NTC in place of R2
Yes.. thank you.. i got the result
OK, glad to help!
I am currently making a circuit for 300mA output current.. can you tell the values of C1, R1 and R2.. i calculated but result is not coming
for 300 mA current, C1 can be a 6uF/400V PPC capacitor, R1 can be 1 Meg 1/4 watt, R2 can be a 10 ohm NTC thermister.
Hello, when i am simulating the circuit online.. i am getting nearly proper voltage after zener diode.. but the current is fluctuating.. can you tell me what should i use after zener so that approx 70 mA is achieved?
Helo, you can probably try adding a 1N4007 diode in the positive line that joins the zener cathode with the capacitor positive, and see if that helps. Use a 1000uF/25V capacitor, if the zener value is 12V
Thank you.. my current fluctuations got rectified but still i am not able to attain 70mA..
How did you measure the current, is it by connecting voltmeter across the output of the power supply? Actually 70 mA cannot be achieved using a 105 capacitor, practically you can get only 50mA from it. You can try adding a 0.47uF/400V in parallel to the 105/400V so that the current increases to 75 mA
Although calculations for the surge limiting resistor indicate a1/4Watt resistor is sufficient, that resistor can at worst case have almost peak mains voltage across it, even though for a very short time. Most 1/4watt resistors are simply not built to withstand such peak voltages and the risk is it will arc over internally should the circuit be repeatedly turned on at the instant of peak voltage. The result is a blackened carburised R2 which will either finally fail short or open. Nothing to do with power dissipation, all to do with the effective volts per metre gradient within the resistor. A physically larger resistor mitigates that risk. Where space permits I use two 1 watt resistors in series, but then I am in a country where the mains is 240 volts. In 110V countries that arcing failure mode is much less frequent.
The issue is compounded by the fact that the breakover , or voltage withstand characteristics of resistors are not often quoted. It’s seen to be all about the power handling and the resistance value.
To illustrate the principle: would anyone feel OK about putting 1000V across an 8.2Mohm 1/4W resistor? The power comes out to be only 0.12Watts so based on power calcs it should be fine. But I’m not sure I would be happy with a kilovolt over an air gap of about 4mm, let alone a 4mm component made of ceramic and metal film.
For this application carbon composition resistors fare better than film types, at least in theory. In practice I have had no issues with using two 1/2 resistors of any kind in series, or 1Watt resistors. Both avoid the arcing problems.
Thank you for the useful feedback….two resistors in series looks a better option to me.
Hi!
Concerning the R2 Resistor, which protects the Zener-Diode wouldn’t it be possible to also use a supressor Diode to protect the Zener-Diode and the circuit behind?
Hi, Yes that can be also included for added protection…
I ever experience build the Transformerless Power Supply. When the circuit is in no load condition, the Zener diode generates very high heat and causes the PCB to turn brown.
I find some circuit designs apply a resistor in front of the Zenor diode. What is the purpose? Please advise.
Yes a series resistor is required otherwise the zener will quickly burn. R2 is specifically introduced for this purpose!
Place write hindi ,so it is easy undertand for transformer less power supply,
Hello dear swagatam
Thank you so much for your very interesting and informative essay. I learned a lot. would you please tell me how to calculate the wattage of R1 and R2? I need to use nearly all 70 mA output current to run a fan that needs 60 mA.
Never forget your kindness
Best regards
Thank you Dear Visse, I have added the required information in the above article, you can check it out.
What If R2 is not provided?
zener diode will burn
Thank you for kind reply….
Hi dear Swagatamx
could you please tell about details of 110-240V AC / 12V DC2Amp power supply, transformer? I need datasheet, component, turns, wire cross action and etc.
please help me sir.
thanks a lot
Hi, I have already answered your question in the previous comment!
Hi,
While analsing the current through the network,why don’t you take the effect of R1 and R2 into account.
Even though the resistor R1 is used for discharging the capacitor C1, it is in parallel with the capacitive reactance Xc??.
Please explain.
Hi, R1 resistance is 1M which is too high, and the current through it will be negligibly small compared to the current through C1, so it can be ignored.
Hi , very good article; please how to calculate NTC resistor for power and voltage?
Thanks, Tomo
Thank you Tomo,
I have posted one article ob NTC which you can read in the following link:
https://www.homemade-circuits.com/using-ntc-resistor-as-surge-suppressor/
Hi I m following u for a long time. I show many a circuits for making a transformerless power supply given by you.i tried a few of ten but theres always a problem of heating or over voltage or undervoltage..I tried every thing ever a 2watt 50 ohms resistor starts heating up. Plz suggest me a circuit using 105/400 capacitor and plz show me how to connect maximum no of leds ( white straw head led 5mm)
Hi, please replace the 50 ohm resistor with a 10 resistor, it will not heat, or you an also replace it with an NTC thermistor for better surge control.
You can connect 90 numbers of 3.3 V LEDs rated at 20 mA in series. Remember to put a 300 V 1 watt zener diode across the +/- of the LED string or after the bridge rectifier
…please do not use a 105/400V for 20mA LEDs, instead use 474/400v or 0.47uF/400V
Sir
Why we are using R1 in the ckt
Please explain
Harsha, it is to discharge the high voltage capacitor safely when the circuit is unplugged from AC. This safeguards the user from getting a painful shock on accidental touching of the input plug pins
Sir
thank u
Film capacitor only limits current
But not voltage
How diode will withstand higher voltage
Harsha, the diodes are rated at 1000 V
Sir
Peak inverse voltage is 1000v
Then how it withstand forwardly
Harsha, Please check this for your information:
1N4007 Characteristics:
Maximum Recurrent Peak Reverse Voltage 1000V
Maximum RMS Voltage 700V
Maximum DC Blocking Voltage 1000V
Average Forward Current: 1.0A
Peak Forward Surge Current: 30A
Maximum Instantaneous Forward Voltage: 1.0V
Maximum DC Reverse Current At Rated DC Blocking Voltage: 5.0µA @ 25°C
Typical Junction Capacitance: 15pF
Typical Reverse Recovery Time: 2.0us
Mounting Type: Through Hole
Operating Temperature: -55°C ~ 150°C
So does it mean that for a 1 WATT LED WITH 350mA Current will use C1 as 7uF/400V
Hi dear swagatam
Thank you so much for your very informative blog. I learned a lot. would you please tell me how to drive 1Watt bead led 3 volt 330mamps 24 hr
thanks
regards
No problem Raghvendra, glad you found the post useful…
I would recommend the very last circuit since it is designed to dissipate minimum heat and will be most efficient.
For the transistor you can use MJE350, and for the zener diode 5.1v 1 watt zener diode
Hi Swagatam. I am repairing a 240v saw. I need to replace the voltage dropper capacitor in the circuit feeding the gate of the triac. Its a smd 0805 ceramic. Any idea of the value. Thanks in advance.
Mark.
Hi Mark, according to the online information this can be a 0.1uF/16V SMD capacitor
Hi Swagatam, thank you for your time, very much appreciated.
Mark
Hello, what values of resistors and capacitors should I use to put in action a break coil (105 vdc, 0.38 Amps) from a main source 220 vac? Thank you in advance!
You can use a 8uF/400V capacitor. Make sure to rectify the output through a bridge diode and capacitor, and also use a 110V zener diode across the output
Dear Swagatham
If I use 224pf/400V capacitor (220VAC, line frequency 50Hz) the output current would be 15.7ma. There is no need for any zener diode (for voltage regulation) and series resistor (for current limiting) for driving a single 20ma RED LED (vF1.8V). Is it correct….?
Maximum howmany 20mA RED LEDs can be connected in series using the above 224pf capacitor ….?
Dear Anil, yes technically that’s correct, but the occasional switch ON surge could soon destroy the LED, therefore a resistor and zener diode is necessary to prevent this.
After rectification through 4 diodes and a filter capacitor, the deisgn will support a minimum of 300 / 3 = 100 nos of RED LED in series.
For this configuration, only a limiting resistor should be enough, although a 300V zener across the supply line may guarantee a better safety to the series.
I need a 120v 20mA ckt. What will be the electrolytic capacitor to reduce the ripple after bridge ?
for 120V you will need a 120V zener assuming the input is 220V.
the electrolytic can be 1000uF/25V
Hi Swagatam,
Firstly let me appreciate your activity.
On the other hand I would ask your opinion regarding a “flame detector circuit” using ionization principle.
It consists in two wire rods placed in a flame ( e.g. burner) connected to the circuit so it detects instantly the fire presence.
Did you have such a project knowledges?
Waiting for your kindly answer,
Sorin
Hi Sorin,
Sorry, I haven’t investigated this theory yet, so it’s not known to me. A more effective method of detecting fire is by detecting smoke or gas.
hi, many thanks for your great explanation of transformer-less power supply
just a question, I will glad if you could help me
I have a motor start capacitor, aluminum body, 450v, 20uF capacitor, something similar to this image
https://encrypted-tbn0.gstatic.com/images?q=tbn:ANd9GcSe0yrPEhBHj-U5kUy7Kjqe9Y7mOSs6wj-yoYpET7U-sgK57iY2ZA
can I use it to make a power supply? according to the formulas, it can provide 1.6 amps at 308v dc, as your explanations, which is very convenient, and if that is possible, can I use something like lm317 to make a variable power supply from it?
again thanks
Hi, thanks very much! Glad you are liked it! Yes, no doubt you can use the shown 20uF/400V capacitor, but the problem with this type of power supplies is the surge current. when you switch ON power the capacitor will act like a short circuit for a few milliseconds which will be enough to the fry the LM317 instantly.
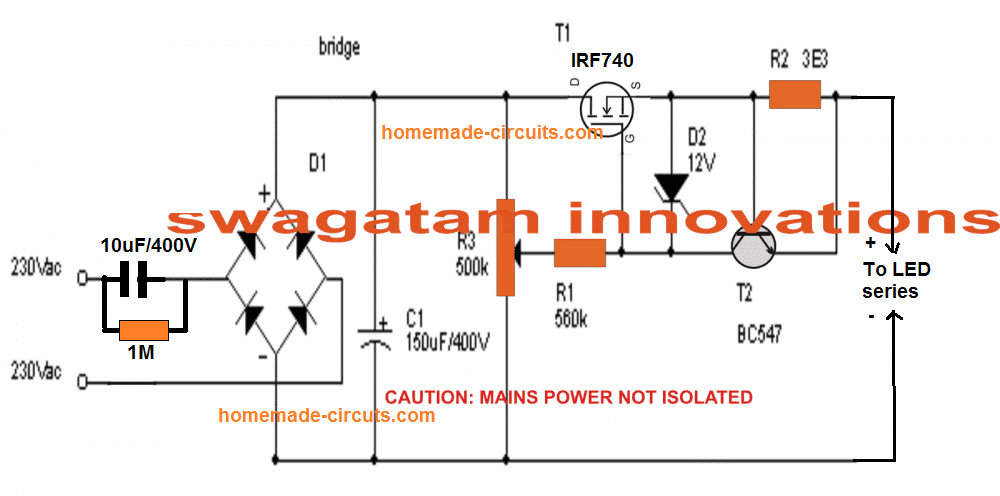
However a mosfet based regulator might do the job, and you can use it as a variable capacitive power supply. Here’s a diagram that you can try:
hi
again thanks for your answer
I just read the pages related to mosfet based regulator transformer-less power supply, and your example provides 350 mA at 310 V dc with a 5 uF capacitor, so with mine 20 uF capacitor I would get 1.4 Amps, right?
if I use this circuit as a variable voltage power supply, I can bring than the voltage for example to 30 V or 12 V via the variable resistor R3 in the attached picture, and of course rest of power ((310-30)*current) must be dissipated through the mosfet heat sink, right again? now my question is that how much current I can get from this circuit at 30 V? can it light a 30 V 30W power led?
Practically a 1uF will give you 50mA output, so 20uF will produce 1 amp.
using mosfet will allow down to 9V, using BJT 2V.
remaining power will be dissipated through heat.
30V 30w is definitely achievable.
Hi Swagatam, How are you ?
I am electronic hobbyist user. I build circuit as given. It is working but R2 (56 ohm 2W) , bridge rectifier and filter capacitor 10uf 250v all component get hot. only change I done is that change value of R2 from 50 ohm to 56 ohm (because I did not get 50 ohm).
Hi Prashant, That looks strange. I can understand R2 getting warm or slightly hot, but the diodes shouldn’t become hot at all because they are 1 amp rated and the 105 cap can produce only 50mA. Similarly with the presence of the 12V zener, the 10uF/250V shouldn’t become hot either, I think there’s some other problem with your circuit, which you must diagnose. Let me know if you have anymore doubts.
good day Sir,,, please i need your help. someone asked me to build automatic power restoration alarm.. please help the circuit diagram thanks
Sunshine, please provide more info regarding the alarm specification.
okay thanks let me try
very nice…good work but sir I need 15v power supply 10A using ferrite core
thank you sunshne, I do not have the design at the moment, but you can try designing it yourself using the following tutorial
https://www.homemade-circuits.com/how-to-design-a-flyback-converter-comprehensive-tutorial/
Hi Swagatam
my question is , is there any difference between placing zener diode before or after the electrolytic capacitor?
thanks
Hi Abba, there’s no difference, both will have the same effect on the output
Hi dear Swagatham,
I have a doubt related to this particular question of Mr.Abba.
I have seen in some Capacitor based transformerless power supplies, the zener diode is connected before the bridge rectifier. If I make more clear my question, consider the above circuit by you, assume ZD1 connecting after C1, R1 junction and the R2. (As per my knowledge, before bridge rectifier, the supply is AC voltage.) If we connect ZD1 before bridge will stabilize the output voltage….?
Thanks in advance.
Dear Anil,
Connecting the zener before the bridge will allow only half cycles of the AC to pass across the output, and will ground the other half cycles, so the output current will become 50% less.
Therefore connecting the zener before the bridge will waste or reduce 50% power.
Hi,
I have done this project using 2.2uF, 100R and 5.1V Zener. i got output voltage perfectly but i got 90V DC after bridge, How can i reduce that voltage??
Hi, you can reduce it either by adding a zener diode across the bridge or an SCR or a power BJT. The SCR version is shown below:
https://www.homemade-circuits.com/scr-shunt-for-protecting-capacitive-led/
Dear sir , Any possibility to design 12 v /30 amp current with x rated capacitors . will it work ? Badly needed for radiology use . ADV thanks for your reply.
Hi Ananad, a 1uF/400V capacitor will give you approximately 50mA current out, so now you can estimate the required value for getting 30 amps
Hai sir, just tell me am i thinking right, i want to use lm2596 to produce 36volt and 3 amps, im planning to give transformerless ac to dc converter as a input of lm2596, i confusing that what is the voltage and current for the input and how do i get from dropping capacitor
Hi, 3 amp is too high for a capacitive power supply…I would advise you to build an SMPS instead
hello dear swagatam , can i use above ckt as power supply for PIR motion sensor ckt
Hi Shankar, I won't recommend it, better to go for a SMPS adapter, your cellphone adapter will do the job nicely
Hi there.. I need to get 5VDc 1A output from 220VAc 60hz.. Please give me the C and R value
1 amp is too high and is not recommended from capacitive circuits.
Hi Sir,
how I will calculate the current limiting resistor of transformerless power supply. Please feedback my question
Hi Joni, It will need to be calculated as per the specifications of the load that you intend to connect at the output
you can use Ohm's law for calculating the parameters
Hi sir.how to known value of C1 R1?
Hi sir!I'm a student.My project is transformerless power supply (220VAC-12VDC). I want to know about how to caculate C1 and R1 by using real formular. Can you help me?
Hi sokleang, you can refer to this article for the details:
https://www.homemade-circuits.com/2015/01/calculating-capacitor-current-in.html
R1 is not important, you can use any resistor above 330K and below 2M2 for this resistor
Sir,
Please explain what type calculation behind "220v X 1.4=308v",why we using and where we get 1.4 here to multiple, any standard or calculation.
Thanking you,
kumaran muthu
Kumaran,
Please research "RMS voltage" you will get answer
OHHH!!! I was wondering the same thing! But you’ve dropped the last two digits, and so it wasn’t obvious what you were explaining (1.414), and you also didn’t state “1.414 RMS” which to some, (like me, is needed). But thanks for this explanition!
hi guys ,, i have done this circuit as you illustrated in the diagram…. but my output is just 1.5v after brige circuit… can u help me with this
Remove the zener and the capacitor and then check
Hello, thanks for the good article. Really usefull. Im an engineer from Venezuela.
I have a doubt. If i want to test different loads, and i short circuit the output (for maximum current scenario) what is expected to happend. I was told that the voltage Will drop. But i don see why.
Thanks in advance.
Glad you liked it, if you short circuit a capacitive power supply output, the voltage will drop to zero without any harm to any devices. It is because the capacitor will restrict current beyond the rated limit causing the voltage to drop.
No you cannot do that, unless the output load operating voltage doesn't match the input mains level, adding caps won't work, rather would become dangerous for the LEDs.