Capacitors connected in series or parallel are very common in electronic circuits. This is done in order to achieve the desired capacitance value and also to make the performance of the circuit accurate. In the following post I have explained how to connect capacitors in series and parallel:
Connecting Capacitors in Series
When we connect capacitors in series, the total capacitance (C) becomes less than the individual capacitance of each capacitor.
The formula for calculating the total capacitance of capacitors connected in series is:
1/C_total = 1/C1 + 1/C2 + 1/C3 + ... + 1/Cn
To connect capacitors in series, you can follow the steps I have explained below:
- For polarized capacitors like electrolytic or tantalum capacitors connect the positive terminal of the first capacitor to the negative terminal of the second capacitor. Then, connect the positive terminal of the second capacitor to the negative terminal of the third capacitor, and keep doing this until you have all the capacitors connected.
- For capacitors which do not have polarity, such as ceramic disc, PPC, MKT etc, simply connect them one after the other without considering the polarity.
- Finally, you will have two terminals remaining across the ends of the series. To measure the value simply connect these ends to a capacitance meter, or use the above formula.
Solving a Practical Example
We can use the above explained formula for solving a practical example, using two capacitors connected in series, as shown in the following image:
Here, we can see two capacitors, one having a value of 10 µF and the other having a value of 20 µF are connected in series.
Let's use the series formula to find out the result.
1/C_total = 1/C1 + 1/C2
1/C_total = 1/10 + 1/20 = 0.15
C_total = 1/0.15 = 6.66 µF
Connecting Capacitors in Parallel
When capacitors are connected in parallel, the total capacitance becomes the sum of the capacitance of each capacitor.
The formula for calculating the total capacitance of capacitors connected in parallel is:
C_total = C1 + C2 + C3 + ... + Cn
In order to connect capacitors in parallel, we simply have to follow the steps I have explained below:
- For polarized capacitors like electrolytic or tantalum, connect the positive terminals of all capacitors together. Next, connect the negative terminals of all capacitors together.
- Once this is done, the common positive and negative terminals ends of the combined capacitors become the input and output terminals. You can connect these terminals to a capacitance meter to get the results, or use the parallel formula to calculate the results.
- For capacitors without polarity such as disc ceramic, PPC, or MKT, you can connect the two ends of the capacitors in common without worrying about any polarity issues.
- Once connected, their common end terminals can be used to measure the capacitance with a capacitance meter, or the above explained formula can be used.
Solving a Practical Example
Now let;s see how we can solve a practical example where two capacitors are connected in parallel.
As shown in the figure below we can see two capacitors, a 10 µF and another 20 µF, connected in parallel.
Let's use the parallel capacitor formula to find the overall value of the above parallel connected capacitors.
C_total = C1 + C2
C_total = 10 + 20 = 30 µF
Solving a Series and Parallel Combination
How would you find the net capacitance value of a combination where both series and parallel connections are used.
Let's consider the following example:
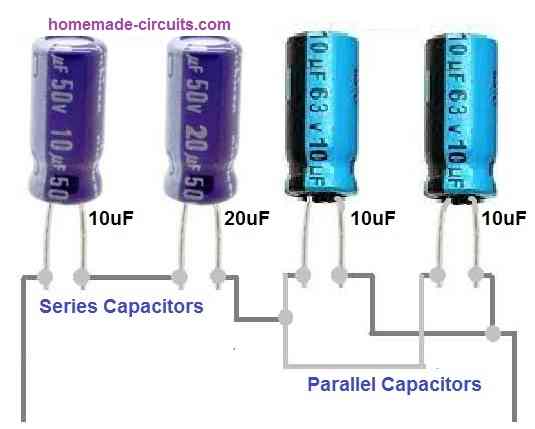
In the above image we can see on the left side a 10 µF and a 20 µF capacitors are connected in series. This series connected is further connected in series with two 10 µF capacitors connected in parallel.
Solving this type of series parallel combination is simple. We calculate the series and the parallel sets separately first, as shown below:
Series capacitors are 10 µF and 20 µF, therefore:
1/C_total = 1/C1 + 1/C2
1/C_total = 1/10 + 1/20 = 0.15
C_total = 1/0.15 = 6.66 µF
Parallel capacitors consists of two 10 µF capacitors, therefore:
C_total = C1 + C2
C_total = 10 + 10 = 20 µF
Thus, the above parallel capacitor becomes a single capacitor of 20 µF.
Similarly, the series capacitors become a single value of 6.66 µF.
Now, it is obvious that these two values are connected in series. Therefore we use the series formula to combine these two values.
1/C_total = 1/C1 + 1/C2
1/C_total = 1/20 + 1/6.66 = 0.20
C_total = 1/0.2 = 5 µF
Therefore, the net value of the above the above series, parallel capacitor combination is 5 µF.
How to Calculate the Voltage Rating of Series Parallel Capacitors
It is actually very simple.
When capacitors are connected in series, you must add their voltage ratings to find the total combined voltage rating of the series string.
When capacitors are connected in parallel, the voltage rating does not change, and remains the same for each capacitor. However, in parallel connection the µF value adds up as is evident in the parallel formula explained earlier.